Perfect Square Dissection
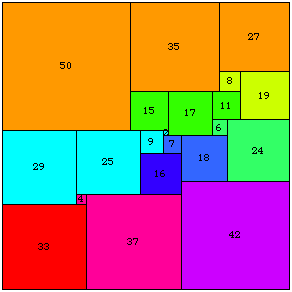
“Can a square be partitioned into a number of smaller squares such that no two of the smaller are the same size? Casual pondering might lead one to think this geometrically impossible. However, in 1939, the first such example was found (R. Sprague, 1939—using 55 squares). Improvements were made by others until, in 1978, it was proven that the “lowest possible order of a perfect square dissection” is 21 (A.J.W. Duijvestijn, 1978).Artist Eric Harshbarger (a graduate of the Auburn University Mathematics Department, 1992 BS., 1994 MS.) has recreated that unique solution in the adjacent mosaic. By using 21 different colors of LEGO bricks, a different color for each square, he has used the popular construction toy to illustrate the geometry of the problem. Asked to explain his motivation further, Harshbarger expounded:
‘The inherent geometry and squareness of the LEGO bricks very much reinforces the underlying theme of the square dissection problem; it is a natural fit. Furthermore, by employing a material more commonly thought of as a popular toy, I hope to draw the audience closer to the work. Too often Mathematics is not considered ‘fun,’ and yet here we see that it is so closely related to one of the most popular toys of all time.’ “