Calculating with large positive integers
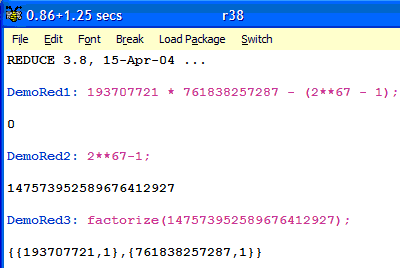
The demonstration version of Reduce for Windows (scroll down page when it loads) – a computer algebra package – can be used to factorise large prime numbers (and polynomials!) as a way of demonstrating the properties of large numbers. Interactive sessions on a projector (the fonts are a bit small but there is no way round that short of altering the projection computer appearance settings) can lead to discussions about primes.
The demo version will not time out, is available free, but cannot be patched and lacks ‘garbage collection’. The latter limitation means that serious symbolic calculations may run out of memory quickly. The demo version arrives as a 29Mb ZIP file. Just unpack the file and drag the folder to a directory and double click on the Reduce icon to start Reduce. A terminal window should appear into which you can type Reduce commands.
The example above shows that the Mersenne number 267 – 1 isn’t prime. An anecdote from Marcus Du Sautoy’s Music of the Primes [ review ] may help to put the calculation into context (my emphaisis)...
“In 1903, Frank Nelson Cole, a professor of mathematics at Columbia University in New York, gave a rather curious talk to a meeting of the American Mathematical Society. Without saying a word, he wrote one of Mersenne’s numbers on one blackboard, and on the next blackboard wrote and multiplied together two smaller numbers. In the middle he wrote an equals sign and sat down.267-1 = 193,707,721 × 761,838,257,287
The audience rose to its feet and applauded – a rare outburst for a roomful of mathematicians…..
... It had taken Cole three years of Sunday afternoons to ‘crack’ this number into its two prime components.”
Computer algebra can take the drudgery out of large calculations, but, as Du Sautoy makes clear, the aim of mathematics is still to understand what is happening. The book provides some insight into why the prime numbers are so important. Chapter 10 goes on to explain the basics of public key cryptography, making the point that there is serious commercial interest in the products of large primes!