[ home ]

Level 1 Adult Numeracy Certificate includes work on fractions. The emphasis is on recognising fraction equivalence, both between proper fractions and between proper fractions and percentages and decimal fractions. Test questions involve finding the value of a fraction of a quantity and converting to and from percentages.
In everyday life, we tend to use certain common fraction families such as halves, quarters, eighths and thirds, sixths, twelfths, and fifths, tenths. Lets face it, fractions with prime number denominators beyond 5 are rarely used except in specialist worlds.
I was looking for a quick activity that could engage everyone. The desks in the classroom are 60cm wide, and 60 is a number with a useful range of factors. I spied some used till rolls waiting for archiving at one of the centres, and that sparked an idea. Till rolls that are about 2cm wide cost about a pound from commercial stationers. One till roll makes enough 60cm lengths for a class of 25 with some left over.
Each student was given a length if till roll ripped off the roll as I went round the classroom, I estimated the length to exceed the width of the desk.
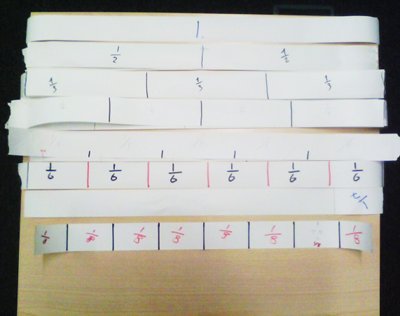
The instructions were to mark the start and end of the 60cm using the desk. Each student was allocated a fraction. They had to divide 60 in the fraction and mark up the roll, and rulers were provided. Students with half, quarter and eighth fractions quickly latched onto repeated folding. Other fraction families needed more figure work, but everyone managed their divisions. Marker pens were given out and students asked verbally to mark each of the fractions on their tape.
When completed, the students were invited to lay their fractions down on the desk at the front as a group. People with the same fractions compared their measuring and put their tapes on top of each other. The idea of ordering the fractions was suggested by a student. Once ordered, I asked questions about the equivalence of the various related families ('how many sixths in a third?') and students pointed out the fact that you could not compare sixths and quarters (for example) directly.
Not a bad use of twenty minutes. Next time, I'll have the instructions on the whiteboard and an example of a marked up tape. And I'll remember the Blu Tak so we can put this on the wall.
Keith Burnett, Last update: Sun Sep 04 2011