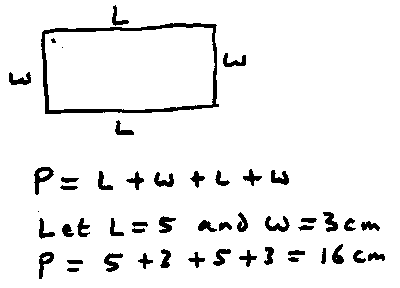
[ home ]
How do I introduce the basics of algebra in a couple of hours to a GCSE class in an adult education centre with quite a wide range of previous Maths education?
Following my use of the area and perimeter of a rectangle to explore factors, I decided to use the perimeter of a rectangle as a vehicle for introducing substitution, collecting terms, simple factorising and setting up and solving equations. My theory is that the rectangle provides a simple visual and familiar model for the algebraic transformations - students can compare what they know about the perimeter of rectangles with the algebraic description. The whole class session below took less than half an hour with plenty of interaction. A futher half hour of individual work on exercises consolidated things.
First of all I draw a rectangle and label the sides with numerical values and ask people to work out the perimeter. Then I ask for different ways of working out the perimeter e.g.
A few numerical examples each using the three methods, everyone gets it.
Now I label the sides with letters instead of numbers.
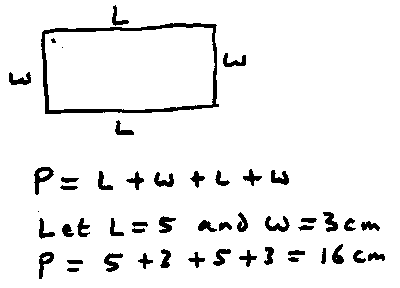
Underneath, I've written the simple P = L + W + L + W formula for the perimeter. Then I have given values to the L and W, and finally, I show that the calculation follows. Letters can take on specific values.
Then we just do a few more really simple substitutions using a few different formulas - just on the whiteboard, not a worksheet.
A new rectangle labelled with L and W and L and W again.
By analogy with the adding up of the two long sides and the adding up of the two short sides, I 'add' the two Ls and the two Ws. Then show substituting some new values into the new formula to link the formula with the calculations during the 'setup'. I also introduce the ≡ sign at this point.
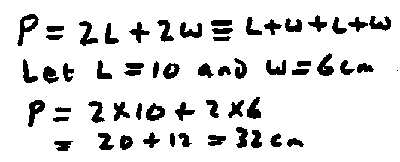
I go through few simple examples of the 2a + 3b + 4a - 2b kind then just to get the idea across. People were taking notes.
Now I bring back the third way of calculating the perimeter of a rectangle, adding the length and the width and doubling the answer. I symbolise this left to right like this...
P = L + W × 2
...and ask what is missing. Brackets are suggested quickly but if not, I fish for bidmas and a question like 'how do you make sure the two sides get added first?'
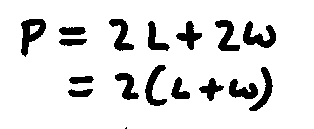
The ≡ sign comes in handy to stress that 2W + 2L ≡ 2(L + W) for any values of L and W including impossible ones like minus a million.
I set factorising a couple of easy expressions like 12a + 9b or 15 + 5x to see if the common factor outside springs to mind. Expanding brackets can also be brought in with 2(L + W) ≡ 2W + 2L. Just a couple of easy positive brackets to expand help to describe the idea.
Now I label the rectangle at having a width of 4cm and an unknown length L. I supply the information that the perimeter is 28cm. Everyone can work backwards to the value of L. Now we symbolise the process...
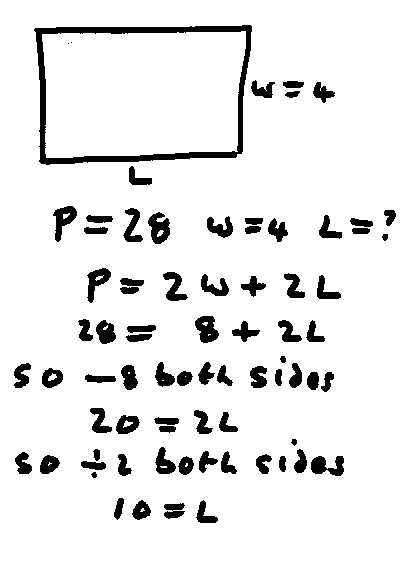
The length of a rectangle is twice its width. The perimeter is 18cm. Find the value of the width and the length.
The length is 5cm more than the width. The perimeter of the rectangle is 42cm. Find the value of the length and width.
I use some 10ticks sheets to consolidate each of the operations, any routine exercises would do. I usually provide answers for my 10ticks sheets and we tend to work down a column.
A bit of directed number practice first, then I set up and used a card sorting task from the TES resources bank. The whiteboard photo below shows an example of one 'row' of the cards.

As you can see the task has three sets of cards, a set of word cards, a set of formula cards, and a set of table cards. The idea is to match the cards. A couple of blank or 'wild' cards are thrown in so students have to formulate a couple of the formulas.
Students managed to match the word cards and formulas, but needed more help with matching the tables as the (usefully) devious author had not shown the values for n=1 in the table. Hints I gave included:
The evaluations were good, students with low confidence on maths generally including tables and calculation found it possible to follow the arguments. I think this may be because of the familiarity of a rectangle and the way the algebraic results can be 'seen' easily. The lesson took very little preparation, just the time taken to select some exercises for consolidation and 'challenge', and the laminating and cutting out of the cards.
Keith Burnett, Last update: Sat Jan 12 2013