[ home ]
Week 2 on the post induction scheme of work at one of my centres is based around multiplying and dividing whole numbers. I usually teach the 'traditional method', grid multiplying and lattice multiplying, then move into short division. I've linked to two the YouTube videos produced by the Manchester based teacher who styles himself 'psychosides', and I play those in the classrooms when I can, sometimes with the sound turned down to see if the students can complete the gaps. The grid or box multiply video is one of my own.
In short division, I look at what the 'remainder' actually means. With some prompting, I can get people to see the remainder as the numerator of a fraction with the denominator being the number thay they are dividing by. Then I extend into decimals, always dividing something smallish by 7 and seeing who notices when the 'remainder' moved to the next column repeats.
This year, I decided to introduce some of the algebra rules early as a bit of a stretch activity as I knew that the basic number work would be tackled quickly. Students had all heard of algebra, and many but not all had specified it as an area they had trouble with at school. The easiest rules to introduce would be the multiplication of terms, just with positive coefficients.
Grid multiplication worksheets (I use the 10ticks level 5 pack) have problems like this...

...students get the hang quickly and there are always a few in each class who like this method as we know from the diagnostics. Those who like the traditional method are encouraged to stick with what they know for general use but to try the grid method a few times.
Then I introduce some puzzles like this
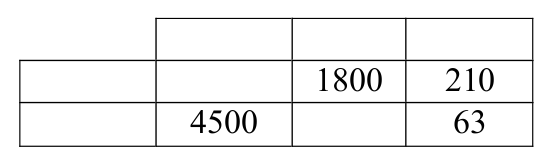
The puzzles start with 2 by 1 grids with the total and one subtotal completed and work up to 2 by 2 grids by the way. I'm presenting 'round the back' problems wherever I can so that students get used to problem solving early (I had the notorious How Tall is the Giraffe Question on the first piece of paper I gave out this year). Once we have discussed strategies for solving these problems, including the powerful constraint that each of the column headings and row headings can only have one digit that is not a zero.
I introduced a small algebra multiplication table...
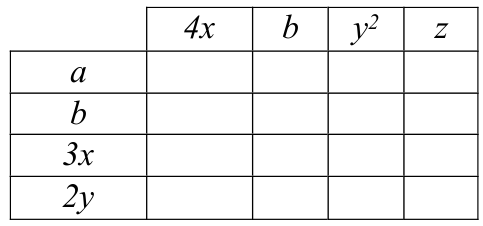
Then, through whole class questions that are open, I built up the algebra multiplication 'rules' as a series of examples
A general recipe: numbers first, then look for repeated letters to sort out the powers, then put the other letters in.
I warned that the textbooks always put the letters in alphabetical order, and that 3yx is the same as 3xy. I also ask about the difference between xy2 and (xy)2.
Then I used the 10ticks pack 6 two sided sheet of algebra multiplication tables. The first side is 'forward' problems which I said where enough at present. The last side is 'work backwards from elements in the body of the grid'. Those were suggested as a challenge.
Next lesson, I'll go over some examples and point out that students have revised index notation and a little bit of sequence of operations as well as their multiplying algebra rules.
Keith Burnett, Last update: Sun Oct 02 2011